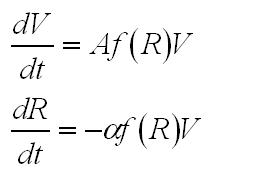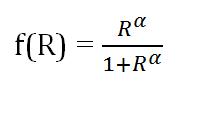Team:Imperial College/Growth Curve
From 2008.igem.org
Prudencewong (Talk | contribs) |
m (Small grammar edits) |
||
| (One intermediate revision not shown) | |||
| Line 4: | Line 4: | ||
{{Imperial/Box2|| | {{Imperial/Box2|| | ||
| - | The first task of our dry lab team was to construct a simple yet effective model for the growth curve of ''B. subtilis''. The inspiration for our model came from the work done by the Imperial College team on the characterisation of Cell Free Systems (<html><a target="_blank" href=" | + | The first task of our dry lab team was to construct a simple yet effective model for the growth curve of ''B. subtilis''. The inspiration for our model came from the work done by the Imperial College team on the characterisation of Cell Free Systems (<html><a target="_blank" href="https://2007.igem.org/Imperial/Cell-Free/Characterisation">here</a></html>) and the elegant manner they found to couple the production terms in the model with the resources available in the medium.|}} |
{{Imperial/Box1|The Model| | {{Imperial/Box1|The Model| | ||
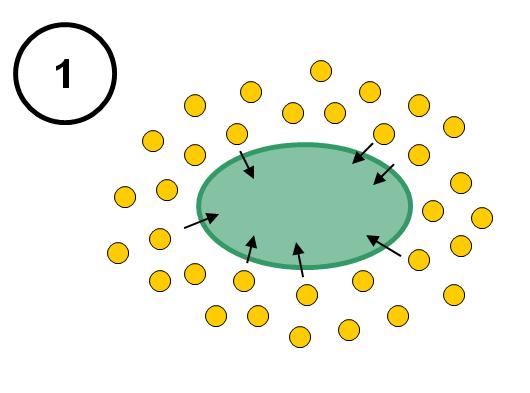
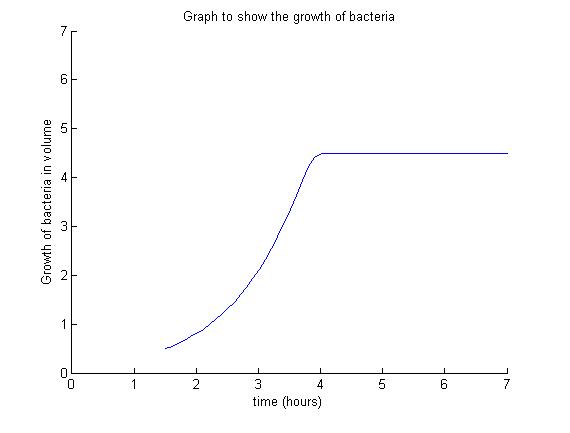
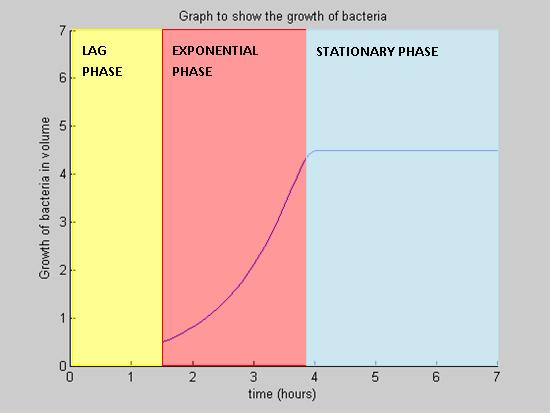
| - | Our model is based on the crucial role played by nutrients ,and other | + | Our model is based on the crucial role played by nutrients, and other resources, during the main growth phases that B. subtilis undergoes - the '''lag phase''', the '''exponential phase''' and the '''stationary phase'''. To keep the model simple, we omit the fourth phase of growth - the '''death phase'''. |
[[Image:Lag_phase.JPG|300px|left]] | [[Image:Lag_phase.JPG|300px|left]] | ||
====== Lag Phase ====== | ====== Lag Phase ====== | ||
| - | During the lag phase, the rate of growth is slow. All nutrients are situated outside the cell initially. Some time is needed for an adequate amount of nutrients to move from the outside of the cell into the interior of the cell. This is vital as the cell requires the nutrients for growth. | + | During the lag phase, the rate of growth is slow. All nutrients are situated outside the cell initially. Some time is needed for an adequate amount of nutrients to move from the outside of the cell into the interior of the cell. This is vital, as the cell requires the nutrients for growth. |
<html><br clear="all"></html> | <html><br clear="all"></html> | ||
[[Image:Exponential_phase.JPG|300px|left]] | [[Image:Exponential_phase.JPG|300px|left]] | ||
====== Exponential Phase ====== | ====== Exponential Phase ====== | ||
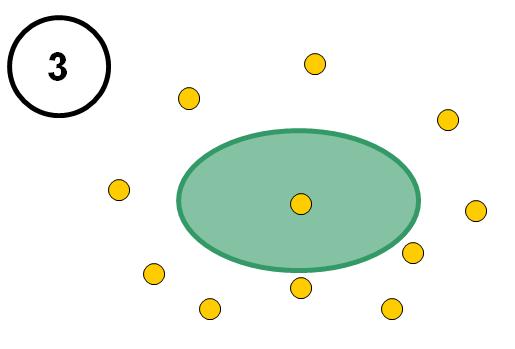
| - | Nutrients are consumed during | + | Nutrients are consumed during cell growth and the growth is exponential as long as there are enough nutrients available. The exchange of nutrients ensures that the intra- and extracellular nutrient concentrations are the same. |
<html><br clear="all"></html> | <html><br clear="all"></html> | ||
| Line 25: | Line 25: | ||
We chose to represent the growth in terms of overall volume rather than number of cells. | We chose to represent the growth in terms of overall volume rather than number of cells. | ||
| - | For small volumes (lag phase and early exponential phase) the volume is a smoother variable (the overall volume grows a little | + | For small volumes (lag phase and early exponential phase) the volume is a smoother variable (the overall volume grows a little in the lag phase) while in later phases, the volume and the number of cells are proportional. <br> |
| - | Considering the overall volume also regularises the process since it allows us, to some extent, to overlook the process of cell division during the bacteria growth. We can | + | Considering the overall volume also regularises the process since it allows us, to some extent, to overlook the process of cell division during the bacteria growth. We can assume the internal concentration of nutrients is reset almost instantaneously to the external concentration. During this time, the total volume does not change. |
<br><br> | <br><br> | ||
| - | In order to couple growth with resources, our model curbs the optimal growth rate of the volume (A) | + | In order to couple growth with resources, our model curbs the optimal growth rate of the volume (A) as a function f of the resources R. We used a Hill function of exponent n, as it models with only one parameter the fact that production is unhindered when resources are plentiful and minimal when resources have run out. Finally we assume that in term of resources the cost of an extra unit of volume is alpha. |
<br> | <br> | ||
| Line 45: | Line 45: | ||
{{Imperial/Box1|Results| | {{Imperial/Box1|Results| | ||
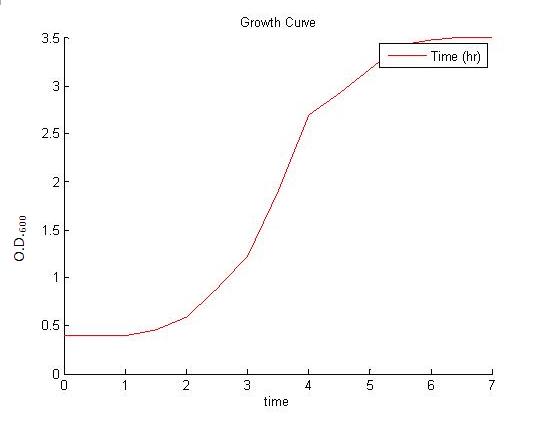
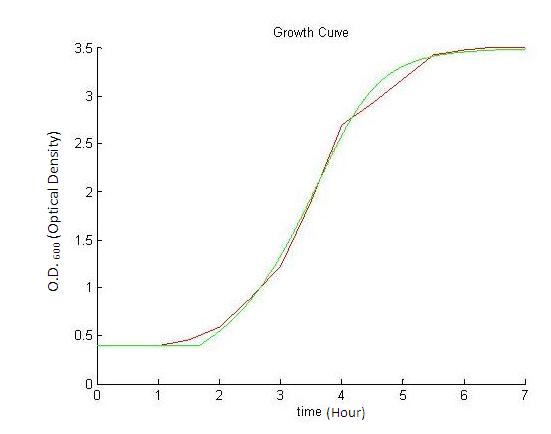
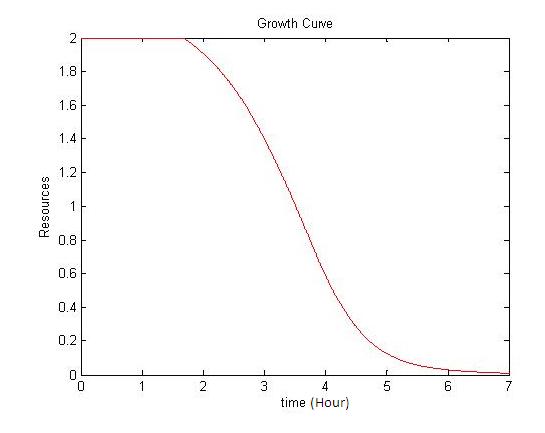
| - | As the simulation below shows, in a rich medium the nutrients are | + | As the simulation below shows, in a rich medium the nutrients are taken up quickly, resulting in a 'nutrient crash'. The transition from exponential to stationary phase is very sudden. |
| - | In the case of our experimental data | + | In the case of our experimental data, the transition is much smoother and the growth far less explosive even in the exponential phase, thus suggesting that the growth medium was not very rich in nutrients and that even at the beginning of the exponential phase the growth was hampered. |
<br><br> | <br><br> | ||
<center>[[Image:nutrient_ft.JPG|350px]][[Image:Label_model.JPG|350px]]</center> | <center>[[Image:nutrient_ft.JPG|350px]][[Image:Label_model.JPG|350px]]</center> | ||
| Line 69: | Line 69: | ||
The initial volume was estimated from the data - likewise the volume in the stationary phase which is directly related to the model parameters R0 and alpha. | The initial volume was estimated from the data - likewise the volume in the stationary phase which is directly related to the model parameters R0 and alpha. | ||
The model parameter A being related to the apparent growth rate of the experimental data, | The model parameter A being related to the apparent growth rate of the experimental data, | ||
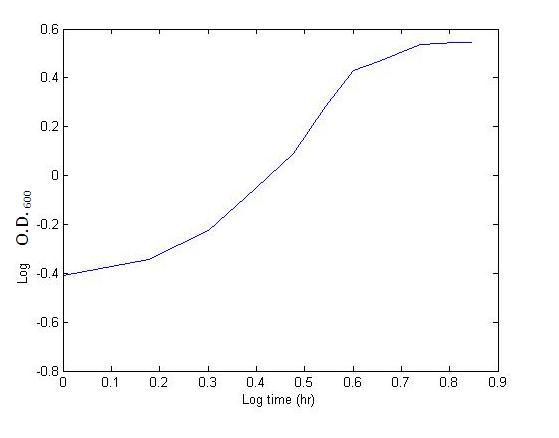
| - | We plotted the log graph to determine the growth rate of our data. | + | We plotted the log graph to determine the growth rate of our data. |
| + | |||
| + | |||
| + | ''Log-Graph used to determine the growth rate'' | ||
[[Image:log_graph2.JPG|300px|Log-Graph used to determine the growth rate|left]] | [[Image:log_graph2.JPG|300px|Log-Graph used to determine the growth rate|left]] | ||
Latest revision as of 04:00, 27 July 2009
Modelling the Growth Curve
|
|||||||||||
 "
"