Team:Imperial College/Genetic Circuit
From 2008.igem.org
m |
m |
||
| Line 44: | Line 44: | ||
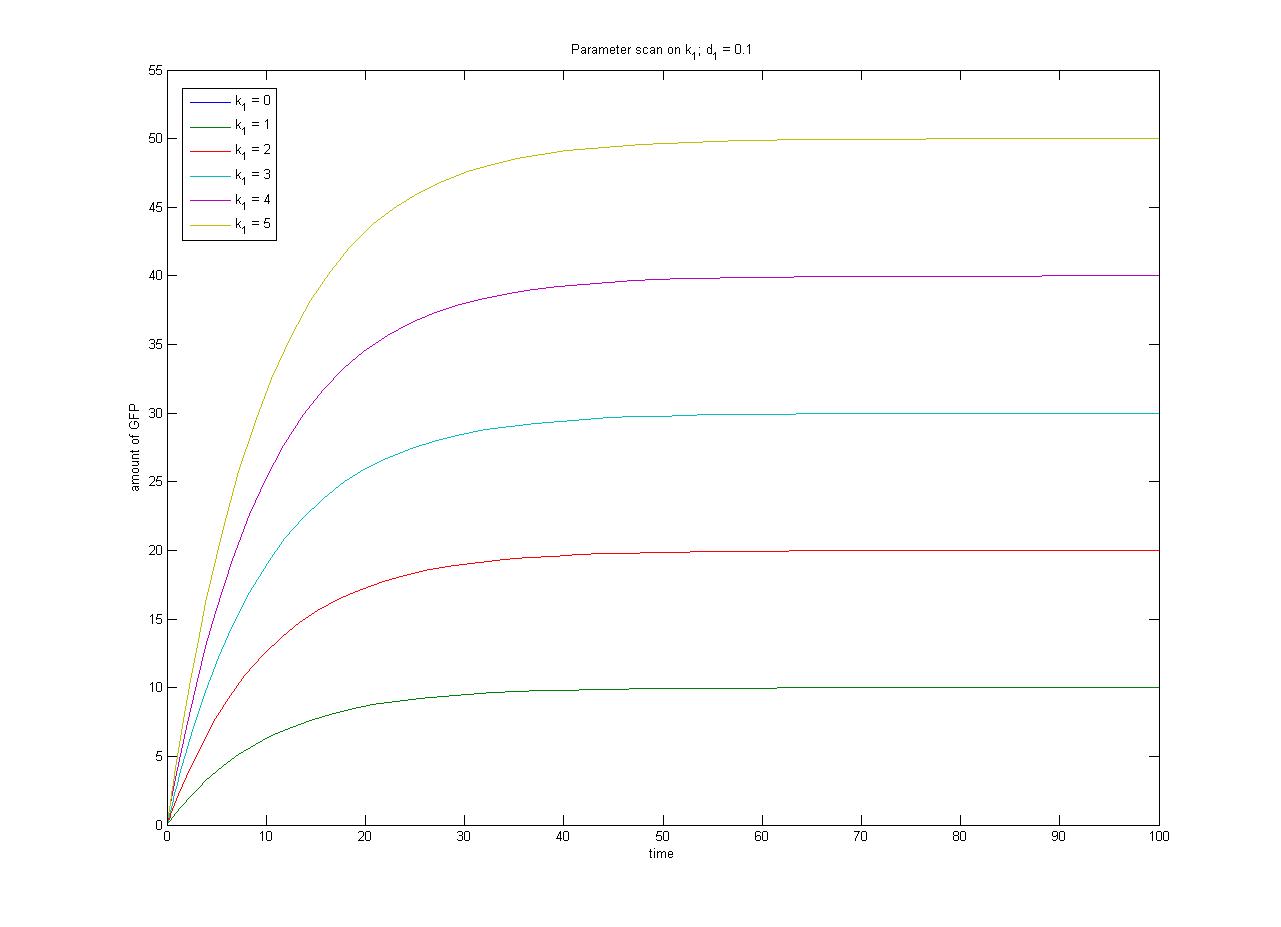
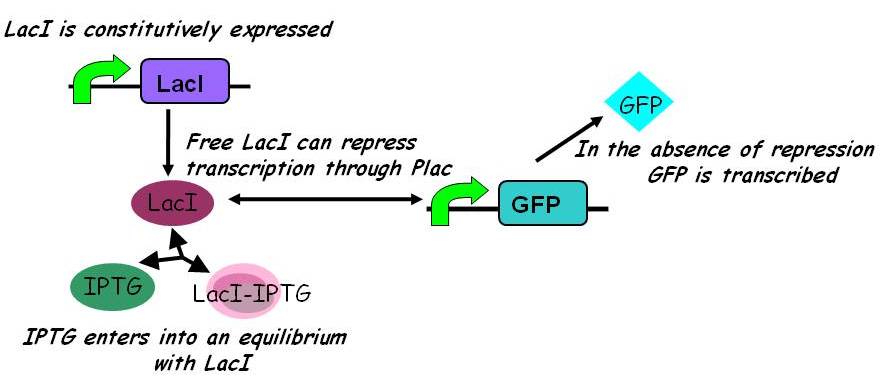
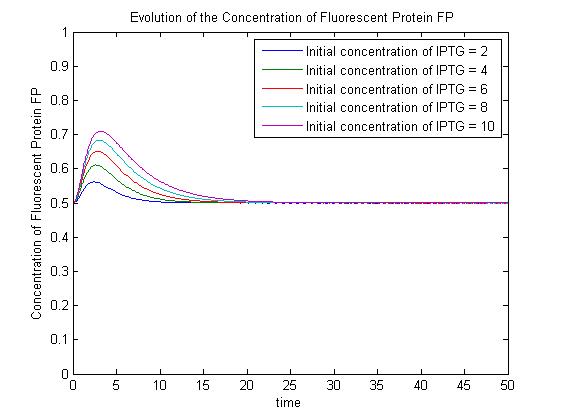
In the simpler model ({{ref|2}}) IPTG competes with free promoter for LacI binding, but does not itself bind to the LacI-promoter complex. [[Click here for more detail]] | In the simpler model ({{ref|2}}) IPTG competes with free promoter for LacI binding, but does not itself bind to the LacI-promoter complex. [[Click here for more detail]] | ||
| - | [[Image:Igem2008 - inducible promoters.jpg| | + | [[Image:Igem2008 - inducible promoters.jpg|435px]] |
Under this model, with all other parameters constant, the steady-state concentration of free promoter and hence the steady-state concentration of GFP are independent of the initial concentration of IPTG. (See time evolution of GFP expression assuming different concentrations of IPTG are used for induction, right.) | Under this model, with all other parameters constant, the steady-state concentration of free promoter and hence the steady-state concentration of GFP are independent of the initial concentration of IPTG. (See time evolution of GFP expression assuming different concentrations of IPTG are used for induction, right.) | ||
| Line 53: | Line 53: | ||
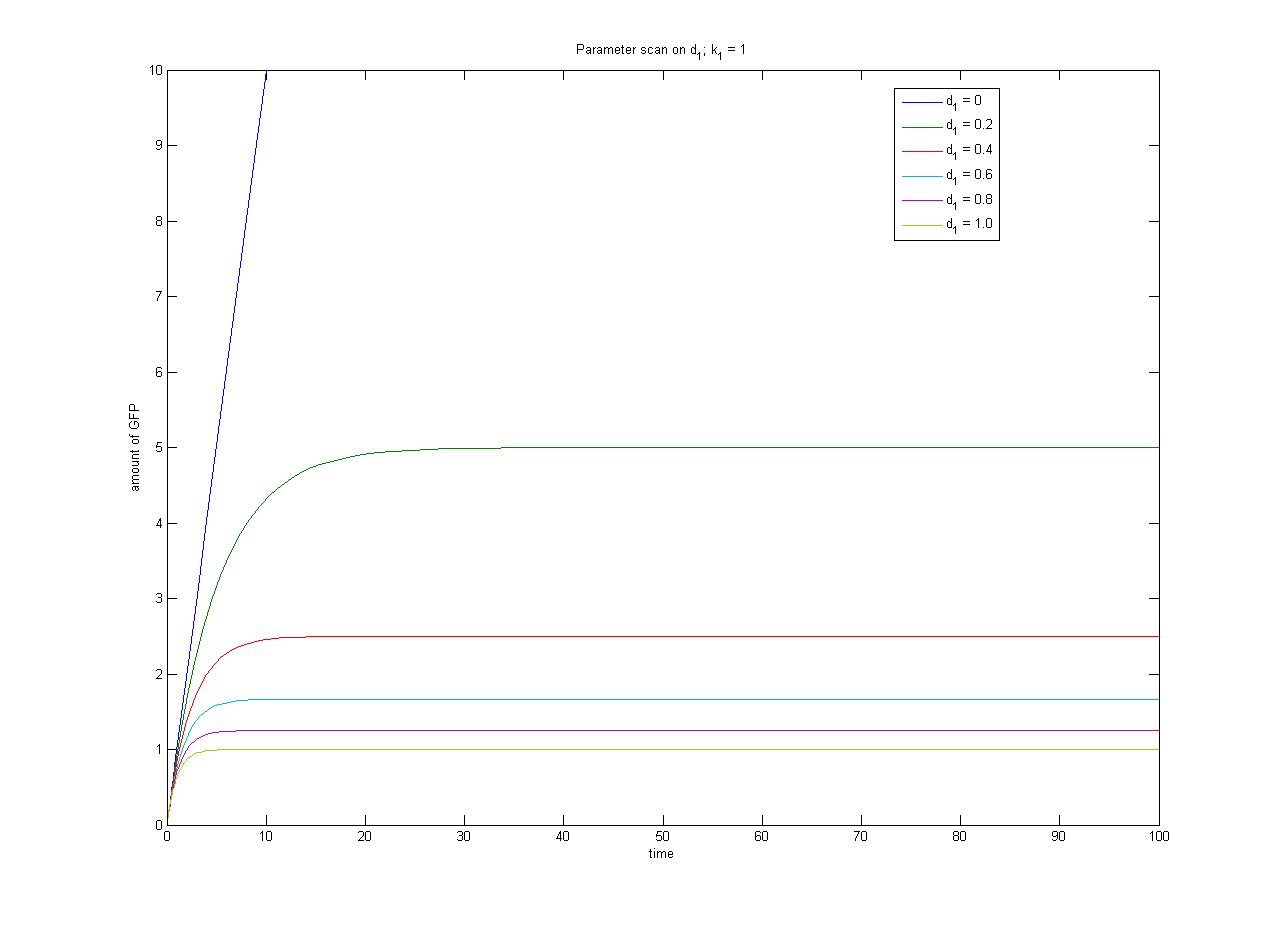
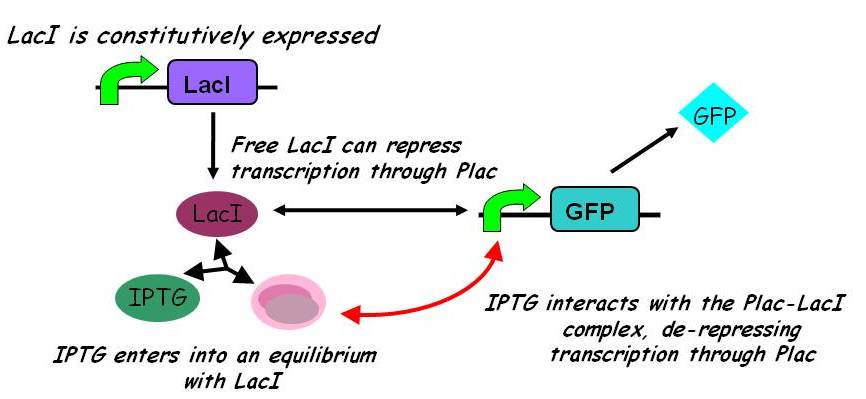
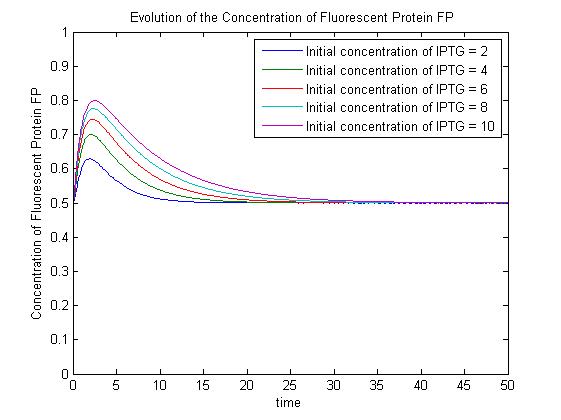
A more sophisticated model allows for interaction between IPTG and the promoter-LacI complex ({{ref|3}}). IPTG can bind to the promoter-LacI complex to form IPTG-LacI and free promoter. This eases the repression by LacI and allows transcription of GFP. | A more sophisticated model allows for interaction between IPTG and the promoter-LacI complex ({{ref|3}}). IPTG can bind to the promoter-LacI complex to form IPTG-LacI and free promoter. This eases the repression by LacI and allows transcription of GFP. | ||
| - | [[Image:Inducible_promoters_2.jpg| | + | [[Image:Inducible_promoters_2.jpg|435px]] |
Under this model, the dynamic behaviour (whether or not [GFP] attains a maximum higher than its steady-state value) depends on the relative strengths of the kinetic constants describing the interactions underlying the model. Either way, the steady-state [GFP] will vary as a Hill-function dependent on the initial concentration of IPTG; this characteristic can be used to discriminate between the two models. | Under this model, the dynamic behaviour (whether or not [GFP] attains a maximum higher than its steady-state value) depends on the relative strengths of the kinetic constants describing the interactions underlying the model. Either way, the steady-state [GFP] will vary as a Hill-function dependent on the initial concentration of IPTG; this characteristic can be used to discriminate between the two models. | ||
Revision as of 02:47, 30 October 2008
Modelling the Genetic Circuit
|
|||||||||||||||||||
 "
"