Team:Imperial College/Motility

As part of our chasis characterisation process, we have decided to model B. subtilis motility. In order to do this, the approach illustrated below was taken. The first phase of modelling involved data collection using microscopy techniques and cell tracking. Collected data was then analysed using algorithms which enabled us to extract distributions of parameters as defined in our model.

Materials

We used the Zeiss Axiovert 200 inverted microscope and Improvision Volocity acquisition software. This system offers a full incubation chamber with temperature control and a highly sensitive 1300x1000 pixel camera for fast low-light imaging. Video images are captured into memory by the system at a basal video frame rate of 16.3Hz. This can be further increased to 27.9Hz by performing x4 binning.

Method

We manually tracked motile B. subtilis, obtaining two-dimensional coordinate data points which describes by the trajectory of the cells. The open source tracking software can be found [http://rsbweb.nih.gov/ij/plugins/track/track.html here].

Data Extraction

The coordinate data obtained was then fed into algorithms to model cell trajectory and motility.

The following mechanical model was developed.

From the figure above, we equate the drag force and flagellum force to obtain:

Solving this first order ODE, we derive an expression for cell velocity:

where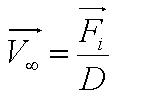

Solving the first order ODE for displacement, we derive an expression for cell trajectory:

Using our fitted data, we are able to determine parameters:

In this model, we attempt to obtain a distribution for the flagellar force, which is represented by parameter A. We assume that the medium is homogenous and the drag coefficient is constant throughout the medium, hence the distribution of flagellar force will be sufficiently be described by parameter A.

Results

The following figure shows the results of our model fitting. We have introduced a change in flagellar force at certain points of the cell trajectory so as to achieve a better fit. A maximum of two runs were allowed for each cell trajectory.

The distribution of parameter A was plotted and modelled according to an exponential model. The following figure describes the probability density function and cumulative density function.