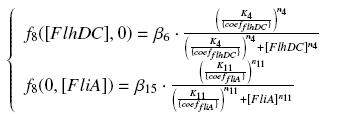Team:Paris/Modeling/f8
From 2008.igem.org
We have [FlhDC] = {coefflhDC}expr(pTet) = {coefflhDC} ƒ1([aTc]i)
and [FliA] = {coefFliA}expr(pBad) = {coefFliA} ƒ2([arab]i)
So, at steady-states,
| param | signification | unit | value | comments |
| [expr(pFlhDC)] | expression rate of pFlhDC with RBS E0032 | nM.min-1 | need for 20 mesures with well choosen values of [aTc]i and for 20 mesures with well choosen values of [arab]i and 5x5 measures for the relation below? | |
| γGFP | dilution-degradation rate of GFP(mut3b) | min-1 | 0.0198 | |
| [GFP] | GFP concentration at steady-state | nM | need for 20 + 20 measures and 5x5 measures for the relation below? | |
| (fluorescence) | value of the observed fluorescence | au | need for 20 + 20 measures and 5x5 measures for the relation below? | |
| conversion | conversion ratio between fluorescence and concentration | nM.au-1 | (1/79.429) |
| param | signification corresponding parameters in the equations | unit | value | comments |
| β6 | production rate of FlhDC-pFlhB with RBS E0032 β6 | nM.min-1 | ||
| (K4/{coeffliA}) | activation constant of FlhDC-pFlhB K4 | nM | ||
| n4 | complexation order of FlhDC-pFlhB n4 | no dimension | ||
| β15 | production rate of FliA-pFlhB with RBS E0032 β15 | nM.min-1 | ||
| (K11/{coefomp}) | activation constant of FliA-pFlhB K11 | nM | ||
| n11 | complexation order of FliA-pFlhB n11 | no dimension |
Then, if we have time, we want to verify the expected relation
 "
"