Team:Imperial College/Genetic Circuit Details

Equilibria

Interactions between IPTG, LacI and free promoter and the formation of the promoter-LacI and IPTG-LacI complexes are described using the following equilibria.

Note: 1:1 stoichiometry has been assumed; this model could be adapted to use different stoichiometric coefficients.

k2, k3, k4 and k5 represent binding constants for formation and dissociation of complexes.

Equations

Before IPTG is introduced the system is at steady-state. The steady-state levels of LacI, free promoter, and GFP are given by:

Note: k1 and k8 represent the rate of transcription through the constitutive promoter upstream of LacI and the promoter upstream of GFP respectively.

d1 and dGFP represent the degradation rates of LacI and GFP respectively.

k_beta is defined as k4/k5.

To describe the change in concentration of the interacting species over time we used the following system of differential equations:

These are evaluated numerically using Matlab's ODE solver.

Note from the differential equations above that the steady-state concentration of free promoter will be independent of the concentration of IPTG introduced into the system. The pre-steady-state maximum concentrotion of GFP attained will differ, however - this is illustrated on the Genetic Circuit page and can be explored further using the simulations.

Equilibria

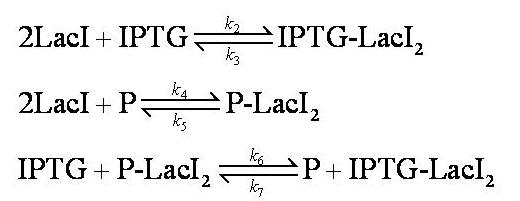
Assumptions:

No intermediary P-IPTG-LacI complex is formed.

We assumed 2 LacI molecules bind to the promoter, in accordance with the model description (2).

Note: k2, k3, k4, k5, k6 and k7 represent binding constants for formation and dissociation of complexes.

Equations

Before IPTG is introduced the system is in a steady state:

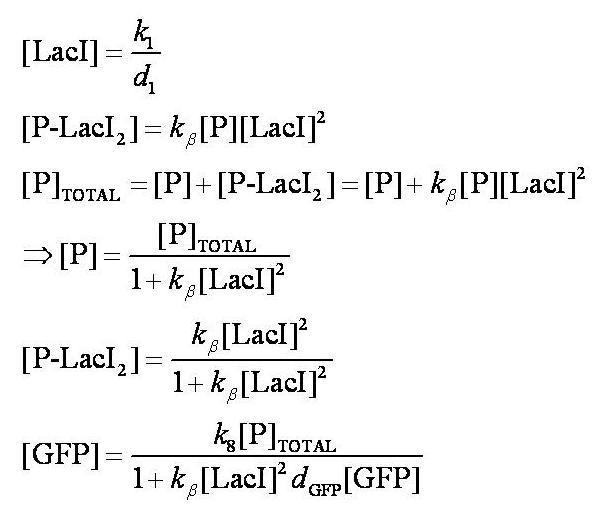
Note: k1 and k8 represent the rate of transcription through the constitutive promoter upstream of LacI and the promoter upstream of GFP respectively.

d1 and dGFP represent the degradation rates of LacI and GFP respectively.

k_beta is defined as k4/k5.

When IPTG is introduced, the dynamic behaviour of the system is described using a system of ODEs.

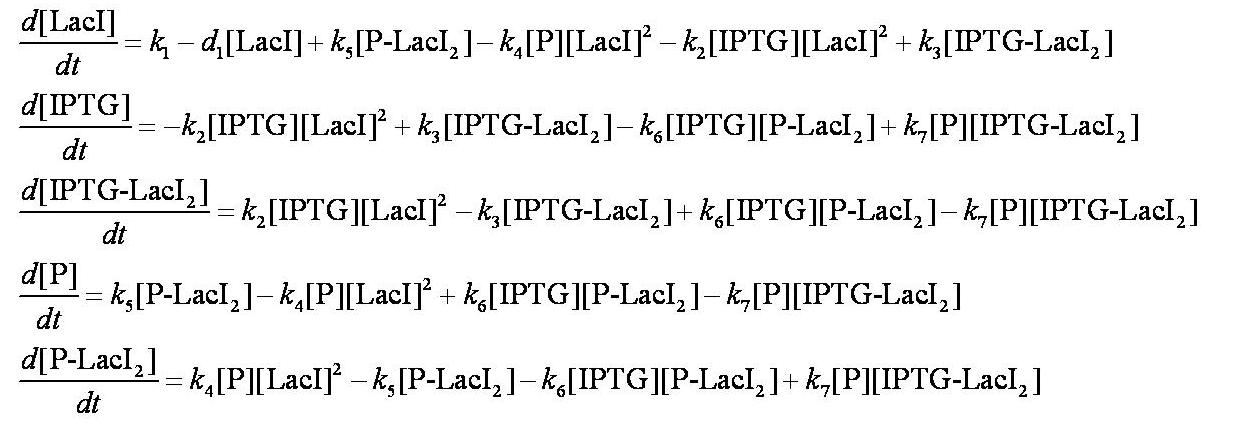
These are evaluated numerically using Matlab's ODE solver.

Qualitative effect of parameters on behaviour

Two different regimes of behaviour can be exhibited by the concentration of GFP over time as described by the more complex model, dependent on the parameters defining the ODE system.

Either the concentration of GFP increases up to a steady-state value, or it displays a hump, increasing to a maximum before returning to a lower steady-state level. This latter case is illustrated on the Genetic Circuit page but both cases can be explored using the simulations.

References

1. Kuhlman T, Zhang Z, Saier MH Jr, & Hwa T (2007) Combinatorial transcriptional control of the lactose operon of Escherichia coli. - PNAS 104 (14) 6043-6048
2. Alon, U (2006) An Introduction to Systems Biology: Design Principles of Biological Circuits - Chapman & Hall/Crc Mathematical and Computational Biology