Team:Paris/Modeling/first parameters
From 2008.igem.org
(Difference between revisions)
(→Set of parameters providing oscillations) |
(→Corresponding code) |
||
| (10 intermediate revisions not shown) | |||
| Line 3: | Line 3: | ||
|-valign="top" | |-valign="top" | ||
|style="background:#ffffff"| | |style="background:#ffffff"| | ||
| + | |||
| + | __TOC__ | ||
==Chosen Modeling== | ==Chosen Modeling== | ||
| Line 19: | Line 21: | ||
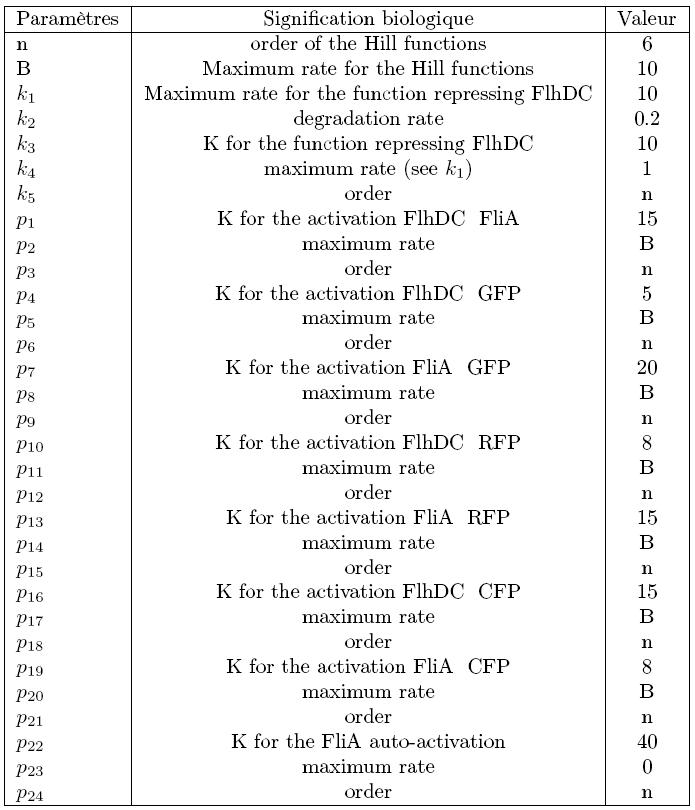
==Set of parameters providing oscillations== | ==Set of parameters providing oscillations== | ||
| - | [[Image | + | [[Image:First_Parameters.JPG|center]] |
| + | |||
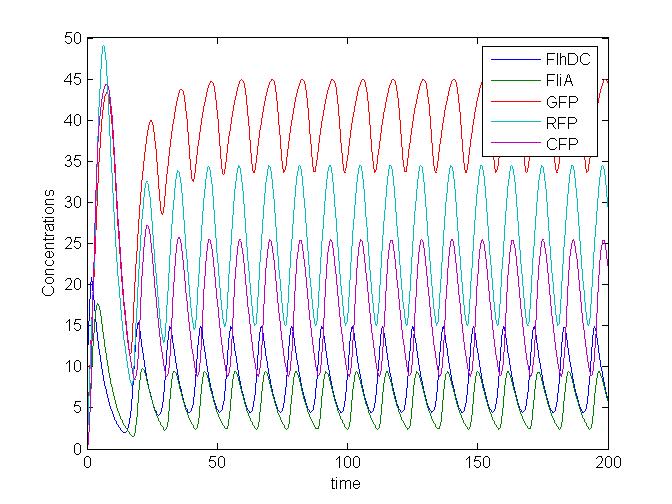
| + | ==Example of oscillations obtained== | ||
| + | |||
| + | [[Image:Fifo_oscill_1.jpg|center]] | ||
| + | |||
| + | Besides, we note that a "First In, First Out" behaviour is obtained concerning the GFP, RFP and CFP concentrations, as shown in the next picture, provided that a gene is 'ON' when it has passed a threshold of 10% of its maximum value. | ||
| + | |||
| + | [[Image:FIFO_1.jpg|center]] | ||
| + | |||
| + | == Corresponding code == | ||
| + | |||
| + | * [[Team:Paris/Modeling/first parameters/premiermodele|First Model]] | ||
| + | * [[Team:Paris/Modeling/first parameters/ODE|ODE]] | ||
| + | * [[Team:Paris/Modeling/first parameters/Simulation|simul]] | ||
|}<br style="clear:both" /> | |}<br style="clear:both" /> | ||
Latest revision as of 16:08, 1 August 2008
|
 "
"