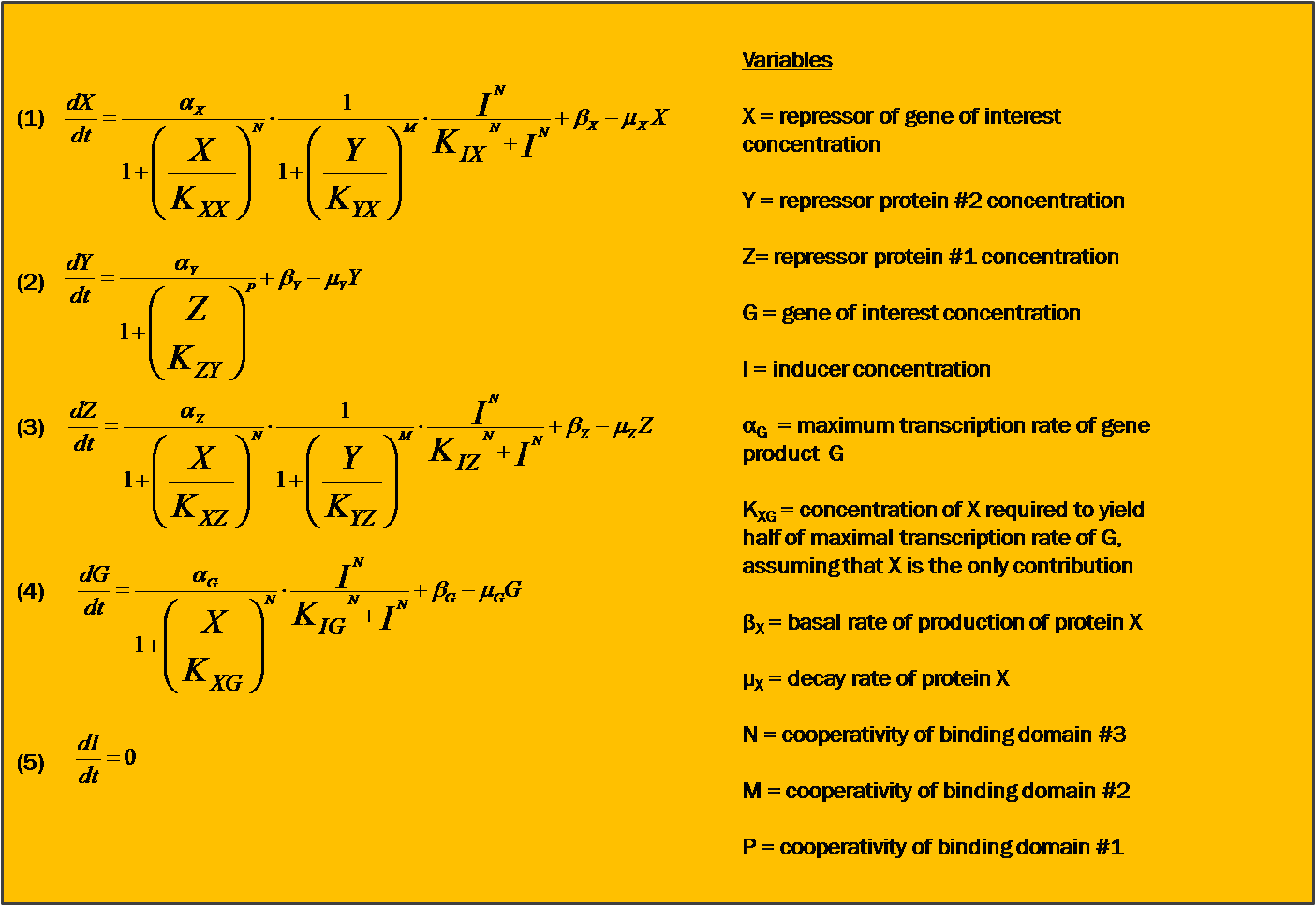
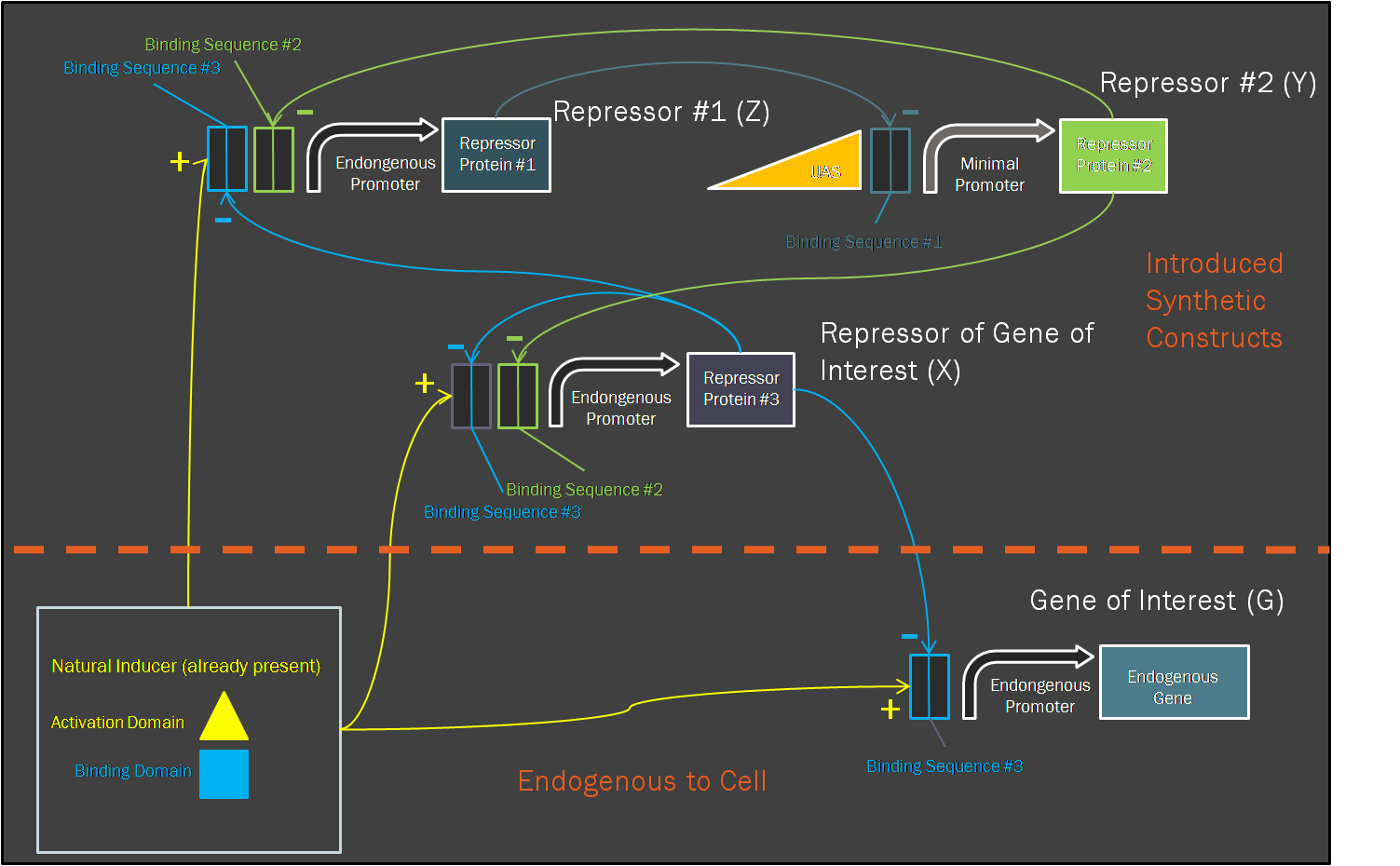
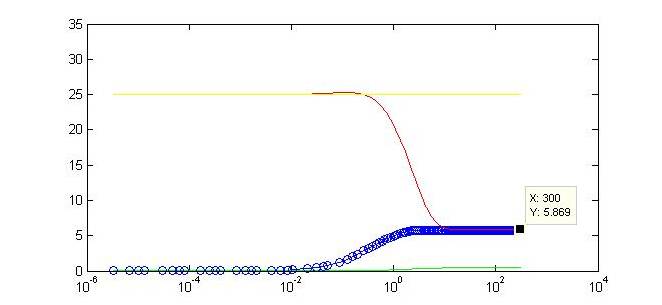
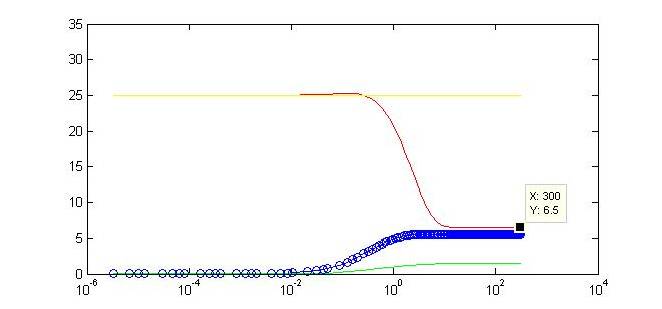
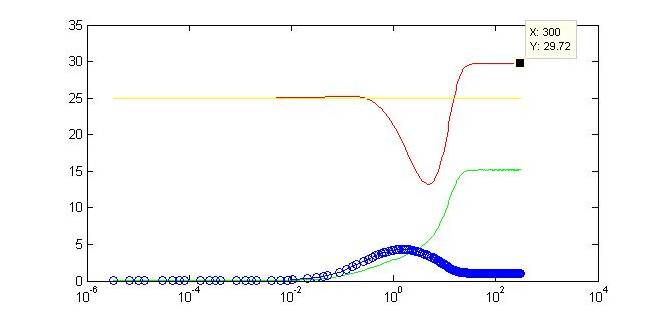
Motivation for Modeling

In contrast to pure experimentation, modeling allows for the rational design of synthetic biological systems. Part characterization makes it possible to apply the known activity of genetic parts to developing a prediction of their qualitative behavior when they are pieced together into a composite device. Such an approach was aptly demonstrated in the paper by Dr. Ajo-Franklin (Ajo-Franklin et al., 2007). They generated a Hill equation model for composite system, to which they applied experimentally-quantified values for the parameters representing the individual parts in the system. Their predictions with this approach matched up considerably well to measurements they made once they have built their final device.

Seeing as we incorporating many of the same parts into our own limiter network, we decided to modify their Hill equations to suit our particular design. It was important for us to model our system because it was necessary to ascertain, given the complexity of the transcriptional interactions, whether the design would generate our desired threshold-driven output.

Hill equation

According to a Hill equation, it is possible to model the relationship between a gene (G) and its transcription factors. The equation varies slightly depending upon whether the transcription factor has an activating or inhibitory effect, and one observes a multiplicative effect if several factors regulate expression of the same gene.

We have modeled all gene products in our system using the following equations:

One will note that the variables correspond to this diagram of our system.

Modeling results

Here we display one of numerous modeling configurations used to understand the behavior of our system. In this situation, we vary the constitutive strength of the promoter controlling expression of gene Y. Specific values have been included below. These values reflect experimental values taken for some of the same synthetic transcription factors.

Initial values: X = Y =Z = 0 I = G = 25

Parameters values:

αX = αZ = αG = 15

αY varies between 0 and 10

All K values = 2.9

All cooperativities = 2.3

All decay rates = 0.5

All basal rates = 0.2

αY = 0

αY = 2.5

αY = 5

αY = 7.5

αY = 10

Not only do we display the regulation of our gene of interest to a certain threshold at the end of our timecourse, but we can observe a tunable threshold as this steady state value changes with varying strengths of the . By tweaking certain biologically-relevant parameters is possible to alter the behavior. The ability to swap in different promoters