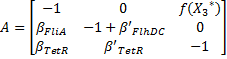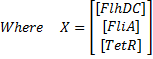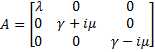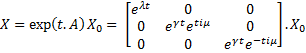Team:Paris/Modeling/BOB/Simulations page travail
From 2008.igem.org
(→Using these elements to improve the system and give directions to the wet-lab) |
(→Understanding the dynamics) |
||
| Line 62: | Line 62: | ||
=== Understanding the dynamics === | === Understanding the dynamics === | ||
* The classical approach consists in finding the equilibrium state, by setting | * The classical approach consists in finding the equilibrium state, by setting | ||
| + | |||
[[Image:Math0.jpg|center]] | [[Image:Math0.jpg|center]] | ||
| + | |||
Then, we evaluate the jacobian matrix, so as to put the system under its linearized form: | Then, we evaluate the jacobian matrix, so as to put the system under its linearized form: | ||
| - | |||
| + | [[Image:Math3.jpg|center]] | ||
| + | <br> | ||
[[Image:Math4.jpg|center]] | [[Image:Math4.jpg|center]] | ||
which gives : | which gives : | ||
| + | |||
[[Image:Math1.jpg|center]] | [[Image:Math1.jpg|center]] | ||
| + | |||
[[Image:Math2.jpg|center]] | [[Image:Math2.jpg|center]] | ||
| - | Then, we want to find the eigenvalues of the jacobian matrix, because they make us understand the behavior of the system. | + | Then, we want to find the eigenvalues of the jacobian matrix, because they make us understand the behavior of the system. Here is the theoretical explanation : |
| + | [[Image:DE1.jpg|center]] | ||
| + | [[Image:DE2.jpg|center]] | ||
| + | [[Image:DE3.jpg|center]] | ||
| + | [[Image:DE4.jpg|center]] | ||
| + | [[Image:DE5.jpg|center]] | ||
| + | |||
expliqeur lexp de matrix puis donner le charpolu puis donner la période | expliqeur lexp de matrix puis donner le charpolu puis donner la période | ||
Revision as of 09:45, 7 October 2008
|
(Under Construction : synchronisation and whole system)
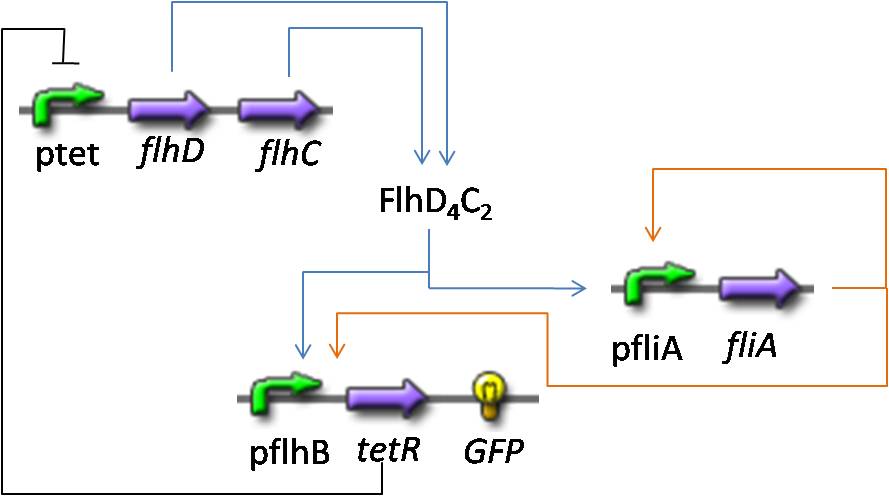
Simulations and Mathematical analysis
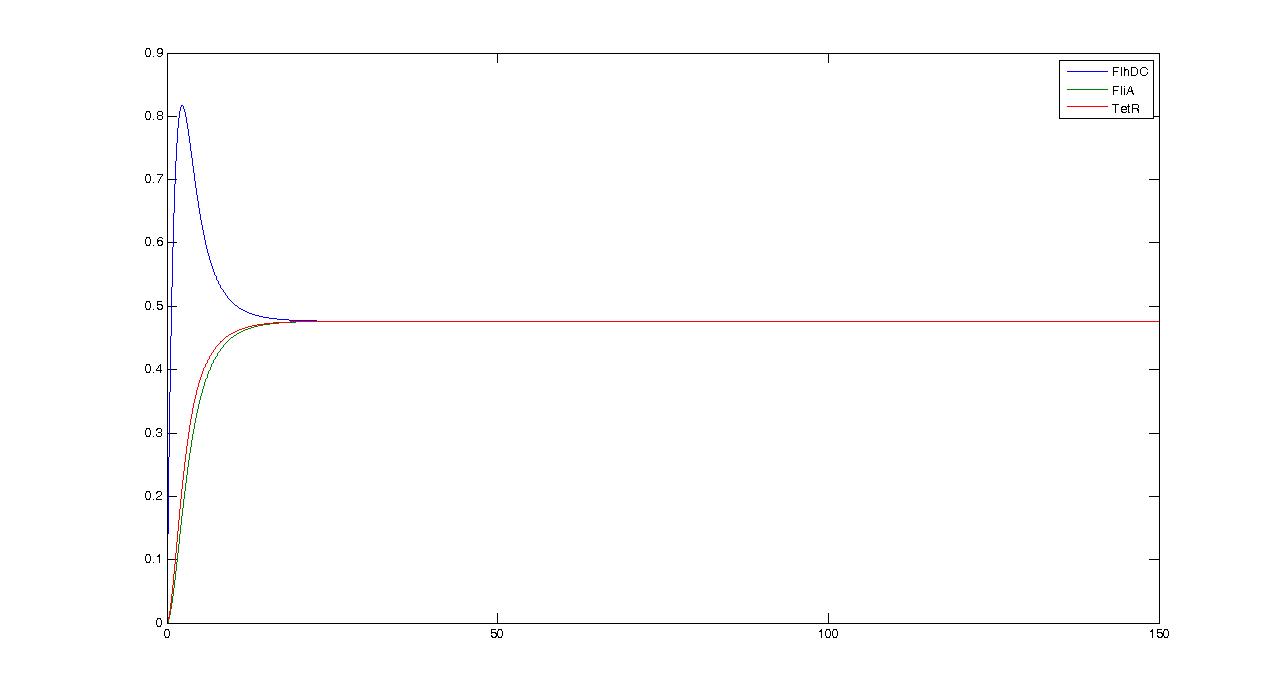
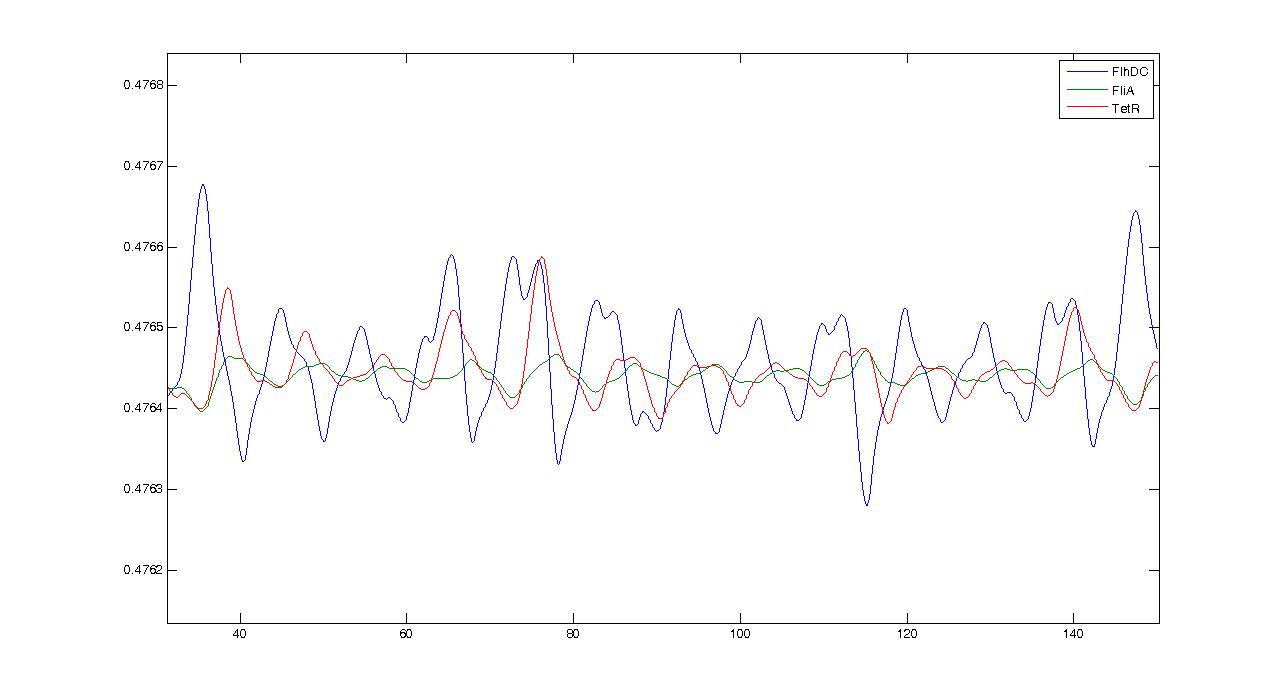
OscillationsShort System
Observations---> petites oscillations + vérifier par le calcul ---> trop grande réactivité de flhDC
Understanding the dynamics
Then, we evaluate the jacobian matrix, so as to put the system under its linearized form:
which gives : Then, we want to find the eigenvalues of the jacobian matrix, because they make us understand the behavior of the system. Here is the theoretical explanation :
Using these elements to improve the system and give directions to the wet-labdire qu'on peut tester les solutions pour obtenir une partie réelle aussi faible que possible, et qu'on peut jouer sur la période au moins dire que pb c'es que mm en dim3, ca devient cho de gérer,mm si c la dim minimale Entire System//biocham ici//+ comparaison avec le modele hillistique FIFO
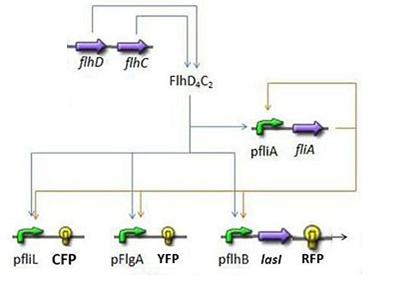
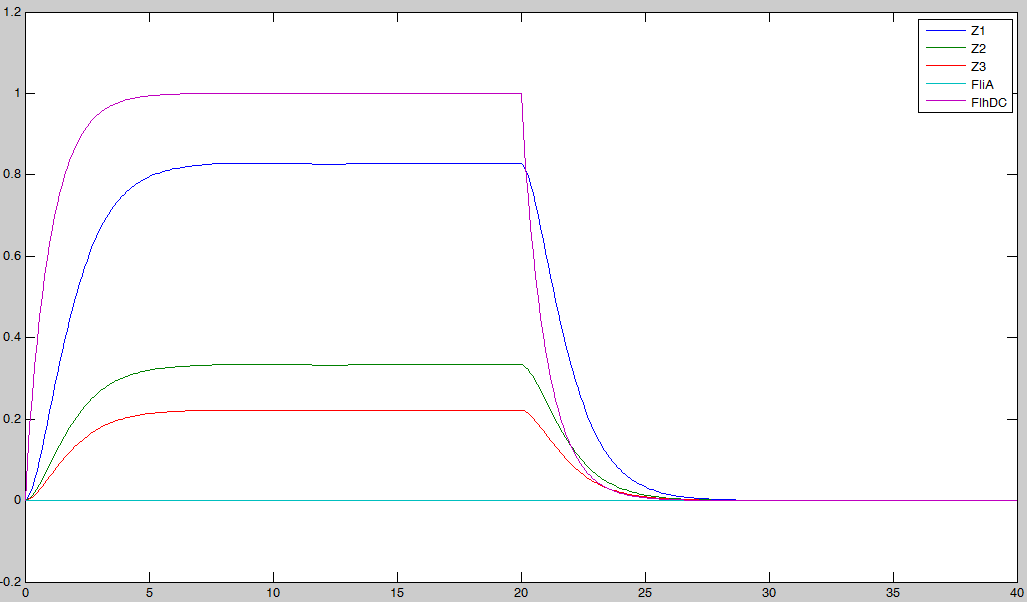
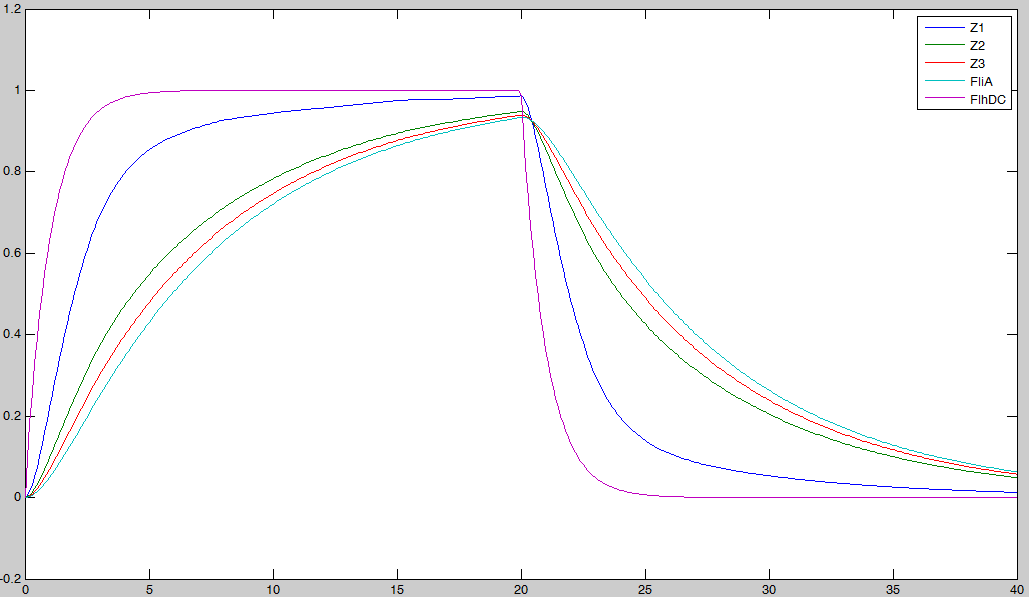
Here is the system we implementated using Matlab (see the corresponding codes) and the corresponding equations (for more detailed information see our establishment of the model). where CFP, YFP, and RFP will be denoted below as respectively Z1,Z2 and Z3.
In fact we assumed that this behavior for FlhDC was acceptable regarding its estimated behavior in the whole system.
We may see that there is a LIFO behavior rather than the FIFO we expect...
SynchronizationMathematical AnalysisSimulations and verification of the hypothesis |
|||||||||||||||||||
 "
"