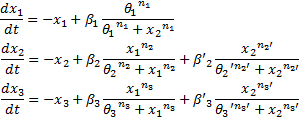Team:Paris/Modeling/BOB/Akaike
From 2008.igem.org
| Line 3: | Line 3: | ||
<center><html><div style="color:#275D96; font-size:2em;">Depending on the experimental constraints, how can we be helped by <br><br>mathematical criterions ?</div></html></center> | <center><html><div style="color:#275D96; font-size:2em;">Depending on the experimental constraints, how can we be helped by <br><br>mathematical criterions ?</div></html></center> | ||
<br> | <br> | ||
| - | * When building a model, it is of the utmost importance to present a justification of the choice made along the transposition process from biological reality to mathematical representation. The aim of this section is to introduce a mathematical justification of our choices in the [[Team:Paris/Modeling/BOB|BOB approach]] since it seems remote from biological reality compared to our [[Team:Paris/Modeling/hill_approach|APE | + | * When building a model, it is of the utmost importance to present a justification of the choice made along the transposition process from biological reality to mathematical representation. The aim of this section is to introduce a mathematical justification of our choices in the [[Team:Paris/Modeling/BOB|BOB approach]] since it seems remote from biological reality compared to our [[Team:Paris/Modeling/hill_approach|APE approach]]. The criterion presented below are to help choosing the most reluctant model '''given the experimental constraints'''. |
<br> | <br> | ||
= Short introduction to the criteria = | = Short introduction to the criteria = | ||
Revision as of 00:25, 23 October 2008
|
Depending on the experimental constraints, how can we be helped by mathematical criterions ?
Short introduction to the criteria
where n denotes the number of experimental values, k the number of parameters and RSS the residual sum of squares. The best fitting model is the one for which those criteria are minimized.
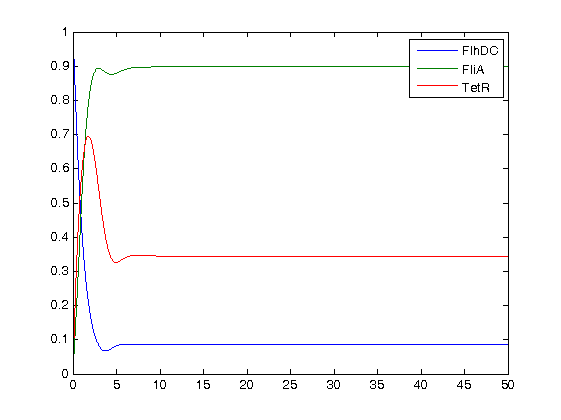
Experiment
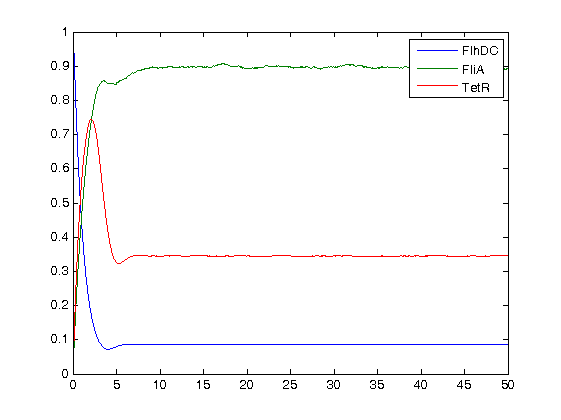
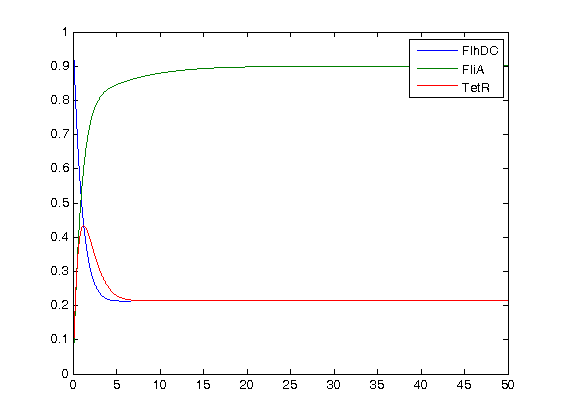
System#1 : using the linear equations from our BOB approach : System#2 : using classical Hill functions :
A fundamental tool?Why can we introduce this seemingly awkard criteria as being a fundamental tool? This precise criteria enables the mathematician to adapt its model. In fact, in that respect, conducting this analysis over his model gives tangible arguments to the mathematician to use such and such model. Indeed, for example in our precise case, if we have about 20 experimental points to fit, BOB approach is sufficient. However, if we get 50 points, BOB approach would be inadequate compared to APE. We believe that this kind of criteria is an essential tool, that might help the model maker to comprehend and control the assumptions he made while creating his model.
[http://www.liebertonline.com/doi/pdf/10.1089/rej.2006.9.324 K. Kikkawa.Statistical issue of regression analysis on development of an age predictive equation. Rejuvenation research, Volume 9, n°2, 2006.]
|
||||||||||||||||||||||||||||||
 "
"