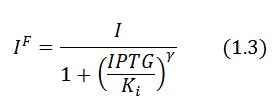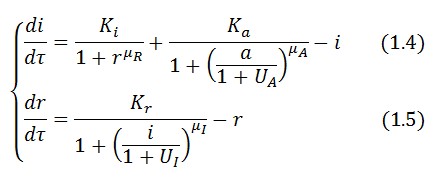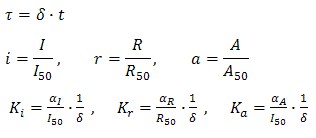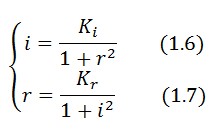"
Team:Bologna/Modeling
From 2008.igem.org
| HOME | TEAM | PROJECT | MODELING | WET-LAB | SOFTWARE | SUBMITTED PARTS | BIOSAFETY AND PROTOCOLS |
|---|
Contents |
Model-based analysis of the genetic Flip-Flop
The genetic Flip-Flop
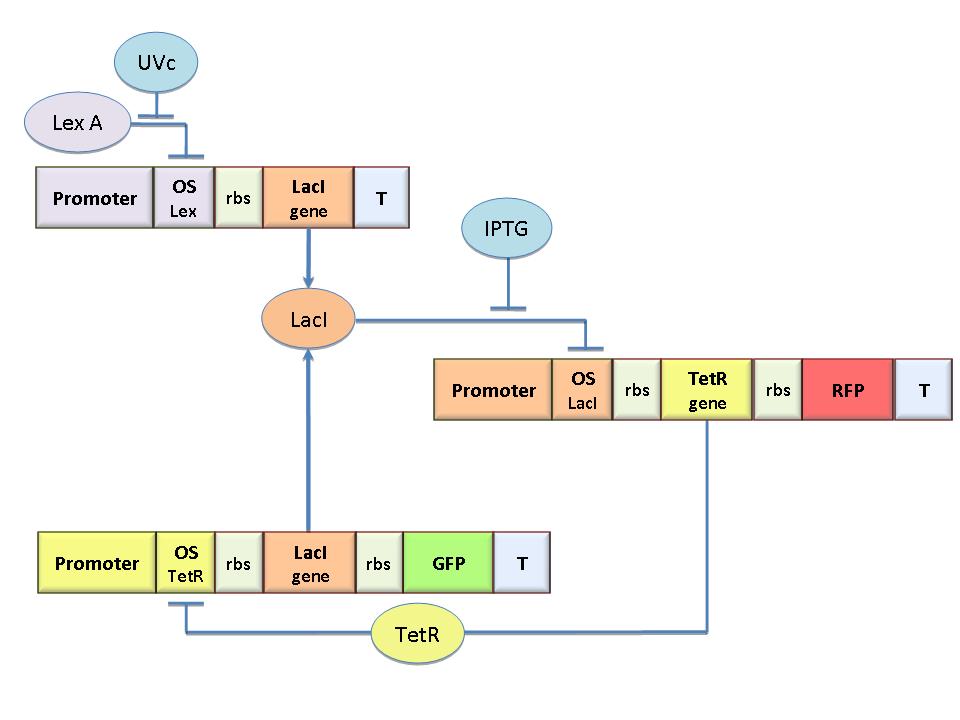
The molecular circuit in Figure 1 works as a Flip-Flop (SR Latch), which can switch between two different states according to the external stimuli (UVc and IPTG).
LacI-ON represents the stable state in which LacI gene is active and LacI protein represses the TetR gene expression, with a positive feedback.
Therefore, the LacI-ON state coincides with the TetR-OFF condition. On the contrary, the TetR-ON represents the state with the TetR gene active and the LacI gene silenced (LacI-OFF). Owing to the coexistence of two stable states (bistability), this circuit is capable of serving as a binary of memory. We denominated it Flip-Flop since it works as a SR Latch: LacI state is the output and TetR state is the output. Uvc is the set signal and IPTG is the reset signal. Indeed, IPTG stimulation inhibits LacI repressor, thus can cause the transition from the LacI-ON state to the TetR-ON, whereas UVc radiation inactiving LexA repressor through the SOS response (Friedberg et al., 1995) can cause the opposite transition from LacI-ON to TetR-ON.
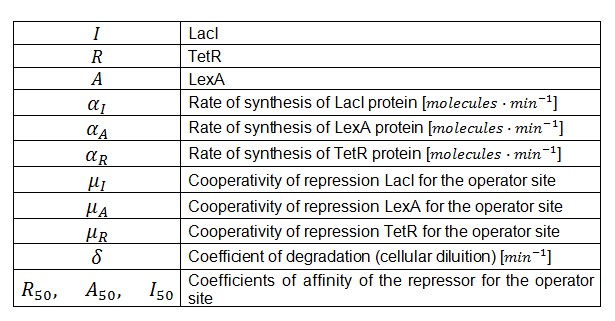
- A common motif in repressor proteins is the presence of a dimeric nucleotide binding site. In accordance to this general structure the cooperativity coefficients () were assumed equal to 2.
In the model we distinguish between LacI protein binded to repressor IPTG 
and protein free 
.
Since 
and considering the law of mass action 
we can write:
Adimensional equations
The equations (1.1) and (1.2) were modified to an adimensional form:
where:
Equibrium conditions
At equilibrium and in absence of stimuli, the adimensional concentrations of LacI (i) and TetR (r) are related by the equation:
To obtain these equations the second term in equation (1.5)was ignored (). This is justified by the high binding constant of LexA for its operator, and the consequent low production of LacI (see Figure 1).
Stability analysis
Assuming as starting condition the LacION state (Figure 1) its stability is guaranteed if the adimensional concentration of LacI is higher than 1 (Figure 2).
In the limit of (), equation (1.7) simplifies:
Experimental identification
The MAVs were identified comparing the experimental response of the genetic circuits in Figure
